THE SHAPE OF REALITY
(Covers "Standard of Learing" items K11, K12; 2.20, 2.21; 3.19; 4.15, 4.17, 4.18; 5.15; 6.14; 8.9, 8.10)

Alternatives to Euclid and the Parallel Postulate

Recall that the Parallel Postulate says (in one of its many forms): Through a given point not on a given line can be drawn only one line parallel to the given line. From this follows that: (1) the sum of the angles of a triangle is 180o, and (2) the Pythagorean Theorem. For many years (literally, for millenia) people tried to prove the Parallel Postulate from more elementary ideas. All attempts failed.

In the early 1800's explorations were made of alternatives to the Parallel Postulate. Big names involved in this include Gauss, Bolyai, and Lobachesvky. To negate the Parallel Postulate, we have two alternatives:

These two postulates lead to two types of noneuclidean geometry: elliptic geometry and hyperbolic geometry, respectively. A special case of elliptic geometry is spherical geometry which is discussed in section 4.6.

Spherical Geometry

A model of spherical geometry is given by considering the "plane" to be the surface of a sphere and "lines" to be great circles on the sphere (great circles are the largest circles which can be drawn on the sphere - they are intersections of the sphere with a plane through the center of the sphere). On the surface of the Earth, lines of constant latitude are examples of great circles.

Question. Are any lines of constant latitude great circles?

We can find the "line segment" (i.e., part of a great circle) through two points on a sphere by putting a string on the sphere that passes through the two points and pulling it tight.

Question. Do two points on a sphere determine a unique "line"?

Question. How many lines are there parallel to a given line?

Now a funny thing happens with triangles when drawn on a sphere - the measure of the angles always add up to more than 180o! If a triangle is "small," then the sum of the measures of the angles is close to 180o (and larger), and if the triangle is "large" then the sum is much more than 180o (see the pictures of triangles on spheres in this section of the text). In fact, we can even have an equilateral triangle with three right angles.

Question. Why does this equilateral triangle appear to violate the Pythagorean Theorem?

Question. How large can the sum of the angles of a triangle be on a sphere?

In fact, may unfamiliar things can happen - the area of a circle is NOT pi time radius squared..

Question. Why is this?

Hyperbolic Geometry

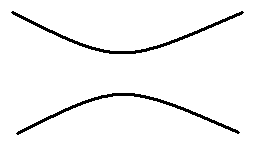
A model of hyperbolic geometry is given by considering the "plane" to be a saddle surface and lines to be strings "pulled tight" on this surface. Pringles chips are examples of such surfaces. Here's a 3-D image of a saddle surface. The "pulling tight" of strings is more subtle here. We must imagine that the string is somehow "trapped" on the saddle and cannot rise up off of the saddle. If we look at the hyperbolic plane then we see that "parallel" lines, due to the curvature of the surface, tend to move away from each other:

Parallel hyperbolic lines
Parallel lines in hyperbolic space.
As a consequence, through a given point not on a given line, there lies an infinite number of lines parallel to the given line:
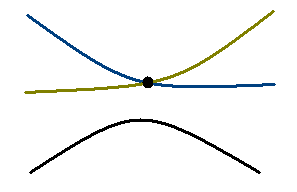
Two lines through a point parallel to a given line
There are multiple lines parallel to a given line
through a given point, in a hyperbolic space.
Since "lines" tend to bend like this, we find that the measures of angles of triangles add up to less than 180o. Small triangles have sums of measures of angles near 180o (but less) and larger triangles have angles with measures summing to much less than 180o (in fact, to 0o in a limit). Here's a 3-D image of a saddle surface with a triangle on it. Again, we find that the Pythagorean Theorem does not hold. Also, the formula area equals pi times radius squared is not valid for the area of a circle.

Curvature

For a path, the curvature reflects how fast the path changes direction. For example, a circle of radius r has curvature 1/r. So a small circle has large curvature and a large circle has small curvature:

Small radius circles have large curvature, large radius circles have small curvature
The curvature of a path can be described in terms of the radii of osculating circles and so is never negative (but the curvature of a line is 0):
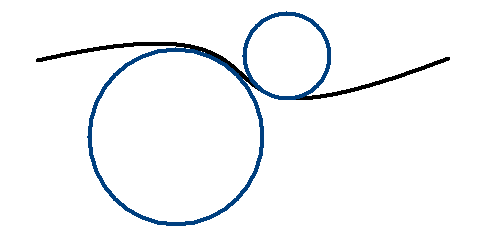
Two osculating circles to a curve
For a surface, curvature can be either 0 (when the surface is flat), positive (when the surface is "puffed out" like a sphere), or negative (when the surface is locally like a saddle - it puffs upwards and downwards at any point). We can discuss this in terms of tangent planes:
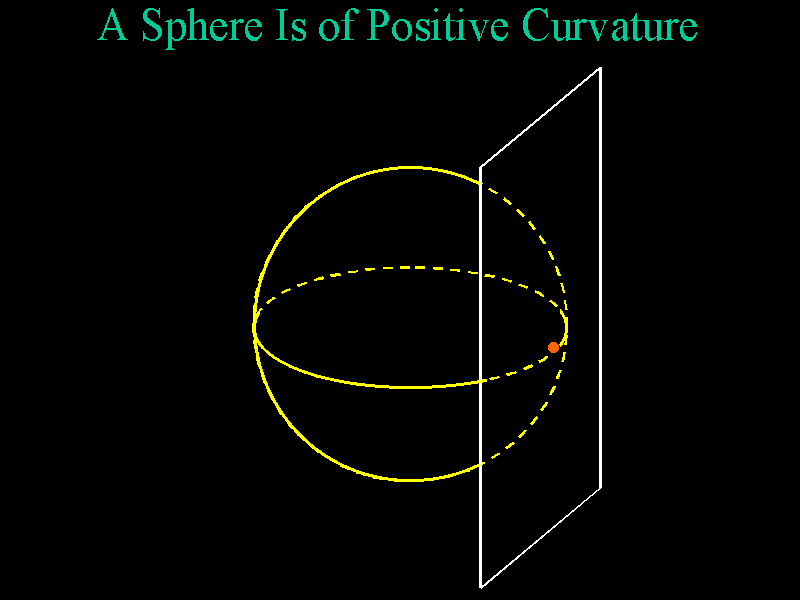
A plane tangent to a sphere
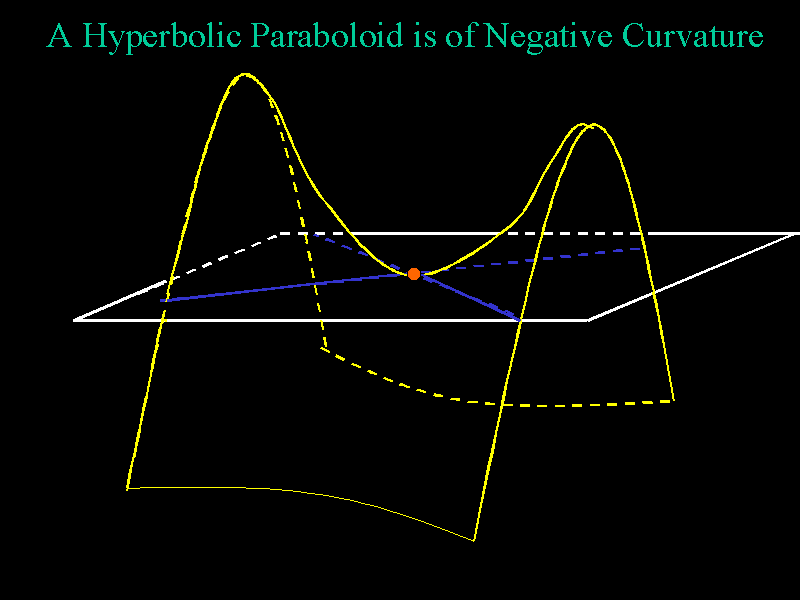
A plane tangent to a saddle-shaped surface

Question. Consider a torus (a donut). Where is the curvature positive? Negative? Zero?

Distances and Metrics

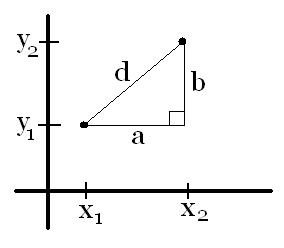
We are used to measuring distances in the usual (Euclidean) way. The distance between points (x1, y1) and (x2, y2) in the Cartesian plane is (thanks to the Pythagorean Theorem)

d = ((x2 - x1)2 + (y2 - y1)2)1/2.
Distance in the Cartesian plane
We know a = |x2 - x1|, b = |y2 - y1|, and so d2 = a2 + b2,
or d = ((x2-x1)2+(y2-y1)2)1/2.
However, there are other ways to measure distance. One way is the "taxicab metric." It is defined as: the distance from (x1, y1) to (x2, y2) is d = |x2-x1|+|y2-y1|:
The taxicab metric in the plane
We know a=|x2-x1|, b=|y2-y1|, and so the distance between the points
under this metric is d=a+b=|x2-x1|+|y2-y1|.
This is a reasonable way to measure distances in a large city where the streets are laid out north/south and east/west.

Question. Are shortest length paths unique under the taxicab metric?

Spider and a Bug on a Wall

We now unite the ideas of the two previous sections. Suppose a spider and a bug are on the walls of a rectangular room. If the spider wants to eat the bug, it must walk along the walls, floor, and ceiling of the room. That is, the spider is restricted to the surfaces of the room (like the "lines" were restricted above). There are multiple paths from the spider to the bug and we want to find the path(s) of smallest distance (using the usual "metric" to measure distance along the surface).

Example. Let's find the shortest path between two points of a 2x1x1 box (or room), by travelling along the sides. We do so by peelling open the room and trying to find the shortest straight line between the points.

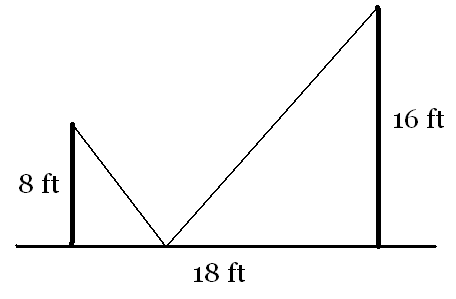
Question. A similar problem sometimes dealt with in calculus is: Suppose a wire is to pass from the top of an 8 foot (vertical) pole, down to the ground, and then to the top of a (vertical) 16 foot pole which is 18 feet from the 8 foot pole. Where on the ground should the wire be attached to be of minimum length?

A calculus problem involving minimum length of a wire

The Shape of Space - Introduction

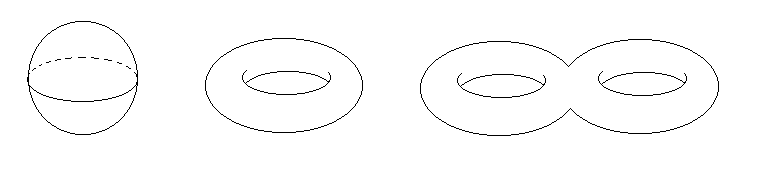
We have discussed surfaces above (spheres, saddles, and boxes). The Earth is effectively some surface, so it is meaningful to ask the question: What is the shape of the Earth? An initial guess might be that the Earth is a flat plane. Are there other possibilities? YES! In fact, one can classify all the possible answers. Assuming the surface of the Earth is "orientable" (more about this latter), then the surface of the Earth must be a some shape in the following pattern:

Orientable surfaces of genus 0, 1, and 2
Of course, the Earth's surface forms a sphere (on the sufficiently large scale - that is, the surface of the Earth is topologically equivalent to a sphere on a sufficiently large scale).

Surprisingly, we can go up a dimension and ask what is the shape of our universe? To start, we could measure the sum of the angles of a triangle to see if it is greater than, less than, or equal to 180o (the book describes such an experiment carried out by Gauss, though this may merely be a legend). Just as we have classified (orientable) surfaces above, we would like to classify (orientable) 3-D spaces so that we would know the list of possible shapes of our universe. This is, it turns out, quite difficult to do! Even with such a list, it may still be hard to empirically determine which possibility actually describes our universe. The text (2nd edition) mentions that NASA's Microwave Anisotropy Probe (MAP) and the European Space Agency's Planck Explorer may provide the necessary evidence. As of now (Fall 2004), our universe appears to be flat with trivial topology (i.e., no surprising connections). To understand what this means, let's further explore surfaces and topological connections...

Return to Bob Gardner's Foundations and Structure of Mathematics 1 webpage.