


Next: Chapter 24
Up: Chapter 23
Previous: Review
- #12
- We are told there are two arms. At 8 kpc, the circumference of the sun's
orbit is
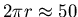 kpc. The distance between the two arms is
25 kpc. Both the sun and arm pattern are moving. The sun is going faster,
so it is constantly overtaking, passing through and moving beyond the arms.
An arm ``sees'' the sun moving at
kpc. The distance between the two arms is
25 kpc. Both the sun and arm pattern are moving. The sun is going faster,
so it is constantly overtaking, passing through and moving beyond the arms.
An arm ``sees'' the sun moving at 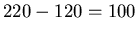 km/s, and so at this relative
speed, it takes the sun a time
km/s, and so at this relative
speed, it takes the sun a time
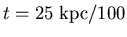 km/s to move from one
arm to the next. This time is
km/s to move from one
arm to the next. This time is
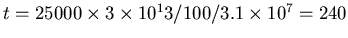 million years. If the sun is 4.6 billion years old (or 4600
million years), it has passed through a spiral arm
million years. If the sun is 4.6 billion years old (or 4600
million years), it has passed through a spiral arm
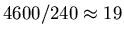 times.
times.
- #13
- The lifetime of an O star, being 10 million years, is smaller than the time
required to move from one arm to the next. It is smaller by the factor
 . The distance between arms is 25 kpc, so at most the O star
can be about 1 kpc away.
. The distance between arms is 25 kpc, so at most the O star
can be about 1 kpc away.
Rico Ignace
2004-09-10