Hoser Film Festival and Research Forum 2 (HFFRF2)
A Failed Attempt!


Bob and Doug McKenzie Figures - The Centerpiece of the HFFRF2
<bgsound src="darkside.wav">
Doug: "(Deep Breath) I am your father, Luke. Give in to the dark side of the force, you knob!"

The second Hoser Film Festival and Research Forum was planned for 2009. However, due to scheduling difficulties, things did not work out. This website describes what was planned. A brief HFFRF2 meeting has been arranged to take place at the 2010 annual meeting of the Southeastern Section of the Mathematical Association of America. It will be held on March 26-27 at Elon University in North Carolina. For the latest details, check out the new HFFRF2 website

The second Hoser Film Festival and Research Forum is again planned for the residence of Dr. Robert Gardner, with Gary D. Coker as the other attendee. The tradition of viewing some of the greatest films of all time with brief episodes of combinatorial research will be continued.

As is tradition, the first film to be viewed will be the inspirational Strange Brew:


Strange Brew
(The original film which inspired the Hoser Film Festival)
A number of other similar genre films will also be presented (such as Batman: The Movie, Young Frankenstein, Blazing Saddles, Top Secret, and for the first time at the HFFRF: The Jerk).

The Research

Following the moderate success of the last Hoser Festival (the eventual publication of a paper in Congressus Numerantium), the continuation of the research component of the HFFRF is expected. Progress has been made in the study of decompositions of complete graphs into copies of the graph H, which is a 4-cycle with a pendant edge. In commemoration of our inspiration, we refer to this as the "Hoser graph."


The Hoser Graph H
Decompositions of the complete graph, Kv, into copies of the Hoser graph (called Hoser systems of order v) were studied by J.C. Bermond, C. Huang, A. Rosa, and D. Sotteau in "Decompositions of Complete Graphs into Isomorphic Subgraphs with Five Vertices" in Ars Combinatoria 10 (1980), 211-254. They showed that a Hoser system of order v exists if and only if v≡0 or 1 (mod 5), v>6.

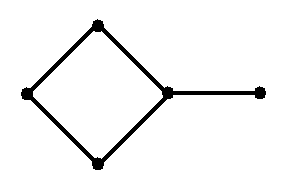
An automorphism of a Hoser system is a permutation of the vertex set of the complete graph on v vertices which fixes the collection of Hoser graphs. An automorphism is cyclic if it consists of a single cycle of length v, and rotational if it consists of a fixed point and a cycle of length v-1. Preliminary research has shown that a cyclic Hoser system of order v exists if and only if v≡1 (mod 10) and a rotational Hoser system of order v exists if and only if v≡0 (mod 10). The initial research plan for HFFRF2 was to address the problem of "bicyclic Hoser systems."

A Hoser system of order v is bicyclic if it admits an automorphism consisting of two disjoint cycles, one of length M and one of length N. As a result of long-distance collaboration (resulting from failed attempts at HFFRF2 from 2007 and 2008), the following has been established:
HFFRF2 Theorem 1. A bicyclic Hoser system of order v, where the bicyclic automorphism consists of disjoint cycles of lengths M and N, where M≤N, exists if and only if

  1. M=N≡3 (mod 10), M=N≥13 or
  2. M≡1 (mod 10), and N=kM where k≡9 (mod 10).
This result has been accepted for publication in Utilitas Mathematica as: "Cyclic, f-Cyclic, and Bicyclic Decompositions of the Complete Graph into the 4-Cycle with a Pendant Edge" by D. Cantrell, G. Coker, and R. Gardner. This paper includes the cyclic and rotational results mentioned above, along with the f-cyclic results of Daniel Cantrell's thesis results.

A New Research Problem

A problem related to those described above concerns the complete graph on v vertices minus a Hamiltonian cycle: Kv - Cv. Decompositions, packings, and coverings of Kv - Cv with the Hoser graph will be the next topic of HFFRF. Automorphisms of each of these are also open problems.

Return to Bob Gardner's webpage. Return to HFFRF1 webpage.
Last updated: March 3, 2010.