I am the author of over twenty-five publications in peer reviewed journals. In addition, I have a published textbook and three encyclopedia articles. A full list of publications can be found in my vita. My primary area of research is in Combinatorics and Graph Theory.
 |
|
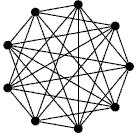
My dissertation was supervised by Robert Jamison. The topic dealt with Labelings and Valuations on graphs and their application to edge decompositions of graphs. For each block of the partition, create a vertex in a the intersection graph. Two vertices in the intersection graph are adjacent if and only if their corresponding blocks share a common node in the original graph. One of the primary questions in my dissertation was to determine when this was possible. One such graph is given below.
Since 2011, my research has primarily focused on games on graphs. In particular, peg solitaire on graphs. Peg solitaire has been played since at least the late 17th century. Perhaps the most famous example of peg solitaire is the 15-hole triangular board found in Cracker Barrel restaurants. My work has generalized this to graphs, hence L-shaped jumps are allowed. Below are some resources on peg solitaire:
- An applet for solving small graphs written by Hal Norwood.
- A list of solvable caterpillars.
- A survey presentation on peg solitaire that I made at Western Carolina University.
- A presentation that I made on Tic-Tac-Toe on Graphs that I made at the MTSU Graph Theory conference in 2015.
-
George Bell has good information on the traditional game.

- Jaap has a nice page with information on a number of puzzles, including peg solitaire.
- Twisty Puzzling has a similar page, complete with videos.
- Ruwix - A wiki for twisty puzzles.
- A presentation that I gave about group theory and the Rubik's Cube.
- A supplement for Abstract Algebra that I wrote about the Fifteen Puzzle.
- A supplement for Graph Theory that I wrote about Instant Insanity.
- The Puzzle Museum has a nice virtual collection of puzzles.
- Stewart Coffin has a nice page on dissection, tiling, and polyominoes.
- Kadon Enterprises is a company that makes puzzles inspired by the work of Major MacMahon (see also this article).
Some good resources for researchers include:
- MathSciNet
- ResearchGate (a social network for connecting to other researchers)
- MathWorld (a math encyclopedia)
- Graph Database (A nice site by Jason Grout)
- LaTeX templates for graphs: TEX, PDF
- The on-line encyclopedia of Integer sequences
- Dynamic Surveys in Combinatorics
- The Grammar According to West
- Getting started with Latex
- The not so short introduction to Latex